In our increasingly interconnected world, we often find ourselves entangled in a myriad of relationships and systems that can be overwhelming to comprehend. It's like navigating through a dense forest, where every tree is connected to another in ways that are not always apparent. Traditional analysis methods sometimes fall short when faced with the depth of these complexities. That's where tools like interrelationship digraphs come into play.
I remember the first time I encountered the concept of interrelationship digraphs. It was during a project management course I took years ago. The instructor, a seasoned professional with decades of experience, introduced us to this tool as a way to visualize and analyze complex problems. At first, I was skeptical. I thought, "How can a simple diagram with nodes and arrows help me understand something as intricate as a large-scale project?" But as we delved deeper into the topic, I began to see the power and versatility of this approach.
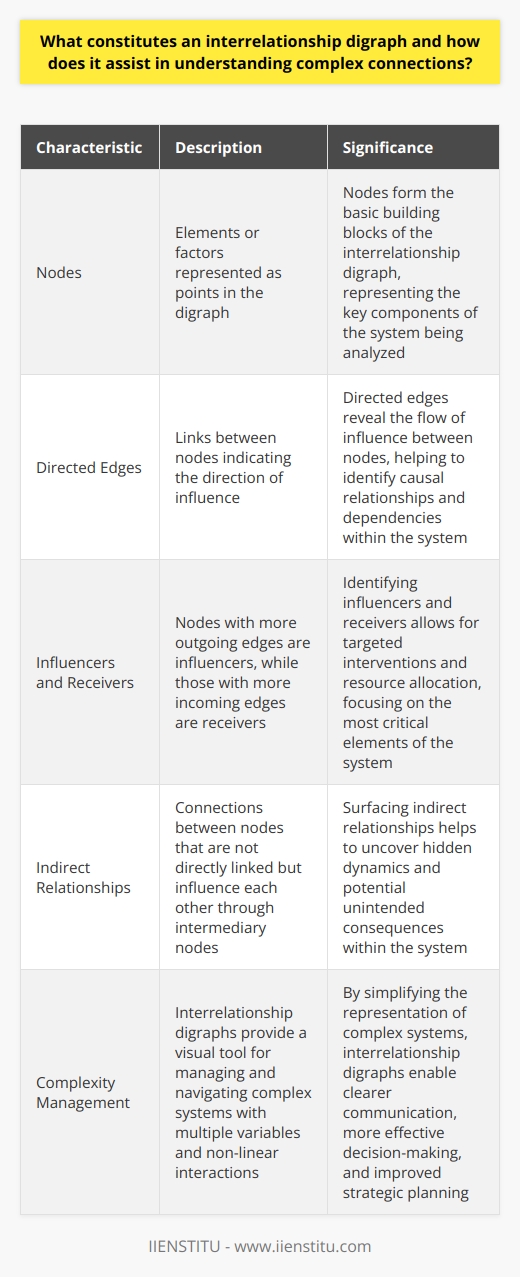
Interrelationship digraphs, also known as directed graphs or digraphs, are visual representations of the relationships between different elements in a system. They consist of nodes, which represent the elements, and arrows, which indicate the direction of influence or causality between these elements. What sets digraphs apart from regular graphs is their emphasis on the directionality of relationships. In a digraph, an arrow from node A to node B means that A influences B, but not necessarily the other way around. This is crucial in understanding cause-and-effect scenarios and identifying key drivers in a system.
One of the key benefits of using digraphs in problem-solving is their ability to reveal hidden connections and dependencies. In complex systems, it's easy to focus on the obvious relationships and miss the subtle, indirect influences that can have a significant impact. By mapping out all the elements and their connections in a digraph, we can uncover these hidden links and gain a more comprehensive understanding of the problem at hand.
For example, let's say you're a teacher trying to improve student performance in your class. You might start by identifying the factors that directly influence student learning, such as teaching methods, classroom environment, and student motivation. However, a digraph analysis might reveal that student motivation is indirectly affected by factors like parental involvement, peer influence, and extracurricular activities. By visualizing these complex relationships with digraphs, you can develop a more holistic approach to enhancing student learning.
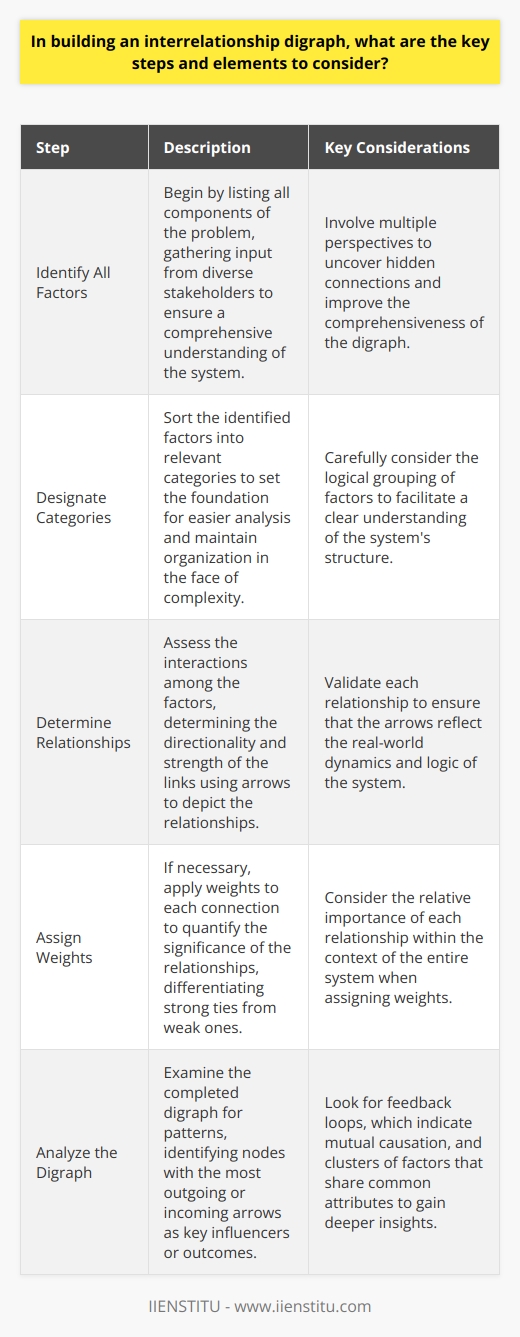
Creating an interrelationship digraph is a straightforward process that can be adapted to any problem or system. The first step is to identify all the relevant elements involved. These could be factors, stakeholders, or components, depending on the context. Next, you determine the relationships between these elements, asking questions like "Does A influence B?" or "Is there a cause-and-effect relationship between C and D?" Once you have established the directionality of each relationship, you can start drawing the digraph, with nodes representing the elements and arrows indicating the direction of influence.
One of my favorite examples of interrelationship digraph application comes from the field of ecology. Ecosystems are incredibly complex, with numerous species interacting in intricate ways. A change in one species' population can have far-reaching effects on the entire ecosystem. By using digraphs, ecologists can map out these interactions and predict the impact of disturbances or interventions. For instance, a digraph of a grassland ecosystem might show how a decrease in the population of a keystone species, like wolves, can lead to an overabundance of their prey, such as deer, which in turn can overgraze the vegetation and alter the landscape. Understanding these digraphs and directed graphs allows ecologists to develop more effective conservation strategies and manage ecosystems sustainably.
Interrelationship digraphs have found applications in various fields, from project management to education, healthcare, and business. In project management, digraphs can help identify critical paths and dependencies, allowing managers to prioritize tasks and allocate resources effectively. In healthcare, digraphs can be used to map out patient journeys, identifying bottlenecks and opportunities for improvement in the care delivery process. Educators can use digraphs to design curriculum maps, ensuring that learning objectives are properly sequenced and integrated.
One of the most powerful aspects of interrelationship digraphs is their ability to visualize feedback loops. Feedback loops occur when the output of a system influences its input, creating a self-reinforcing or self-correcting cycle. Positive feedback loops amplify change, while negative feedback loops counteract it. In a digraph, feedback loops appear as circular paths, where an element indirectly influences itself through a chain of other elements. Identifying these loops is crucial for understanding the dynamics of a system and predicting its behavior over time.
For instance, consider the feedback loops in climate change. As the Earth's temperature rises due to greenhouse gas emissions, it causes ice caps to melt, reducing the planet's albedo (reflectivity). This, in turn, leads to more solar energy being absorbed, further increasing the temperature. This positive feedback loop accelerates the warming process, making it harder to mitigate climate change. By visualizing these loops in an interrelationship digraph, policymakers and scientists can develop more effective strategies to break the cycle and address the root causes of the problem.
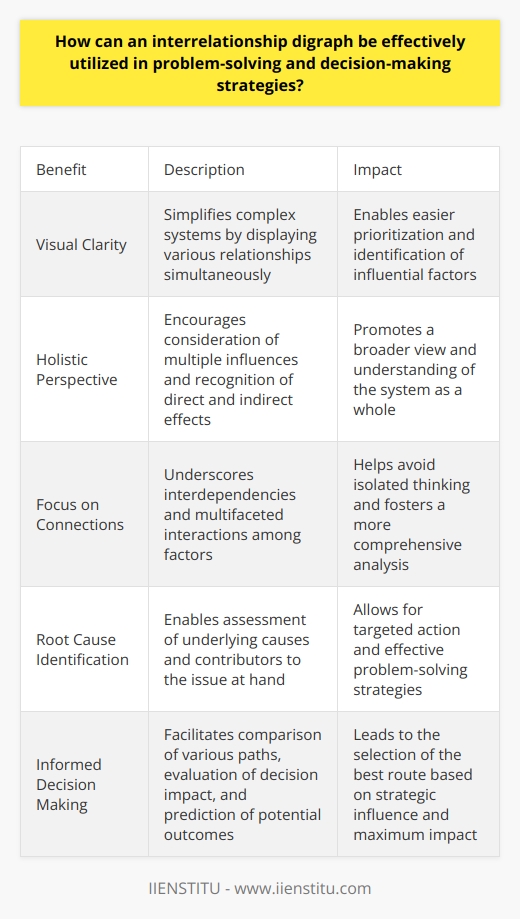
Interrelationship digraphs are not limited to academic or professional settings. They can be valuable tools for personal decision-making and problem-solving as well. Whenever you face a complex decision or challenge, mapping out the factors and their relationships can provide clarity and insight. It can help you identify the key drivers of the problem, anticipate unintended consequences, and develop more robust solutions.
I once used an interrelationship digraph to navigate a career transition. I was feeling stuck in my job and wanted to explore new opportunities, but I wasn't sure where to start. By creating a digraph of my skills, interests, values, and potential career paths, I was able to see the connections and trade-offs between different options. It became clear that my passion for writing and my experience in marketing could be combined into a role in content strategy. This insight gave me the confidence to pursue a new direction and ultimately led to a fulfilling career change.
In today's data-driven world, interrelationship digraphs are evolving to handle larger and more complex datasets. Software tools and algorithms can now generate digraphs automatically from vast amounts of data, uncovering patterns and relationships that might be invisible to the human eye. These advancements have opened up new possibilities for using digraphs in fields like bioinformatics, social network analysis, and strategic decision-making.
However, it's important to remember that interrelationship digraphs are not a silver bullet. They are a tool for understanding and analyzing complexity, but they do not replace critical thinking and domain expertise. Digraphs can reveal relationships and patterns, but interpreting these insights and translating them into actionable strategies requires human judgment and creativity.
Moreover, creating an effective interrelationship digraph requires careful consideration of the scope and boundaries of the problem. It's easy to get lost in the details and include every possible factor, but this can lead to a cluttered and confusing diagram. The key is to focus on the most relevant and influential elements while maintaining a manageable level of complexity. This often involves iterating and refining the digraph as new insights emerge.
In conclusion, interrelationship digraphs are powerful tools for navigating the complexities of our interconnected world. They provide a visual framework for understanding the relationships and dependencies between elements in a system, revealing hidden connections and feedback loops. By applying digraphs to problem-solving and decision-making, we can gain clarity, anticipate unintended consequences, and develop more effective strategies.
Whether you're an educator looking to design engaging curricula, a healthcare professional seeking to improve patient outcomes, or a business leader making strategic decisions, interrelationship digraphs can be a valuable addition to your toolkit. They encourage systems thinking, foster collaboration, and promote a more holistic approach to problem-solving.
So the next time you face a complex challenge, consider grabbing a pen and paper (or a digital tool) and mapping out an interrelationship digraph. You might be surprised by the insights and solutions that emerge when you visualize the unseen connections that shape our world.
References:
1. Capra, F. (1996). The Web of Life: A New Scientific Understanding of Living Systems. Anchor Books.
2. Checkland, P. (1999). Systems Thinking, Systems Practice: Includes a 30-Year Retrospective. John Wiley & Sons.
3. Meadows, D. H. (2008). Thinking in Systems: A Primer. Chelsea Green Publishing.
4. Senge, P. M. (2006). The Fifth Discipline: The Art & Practice of The Learning Organization. Currency.
5. Warfield, J. N. (2006). An Introduction to Systems Science. World Scientific Publishing Company.